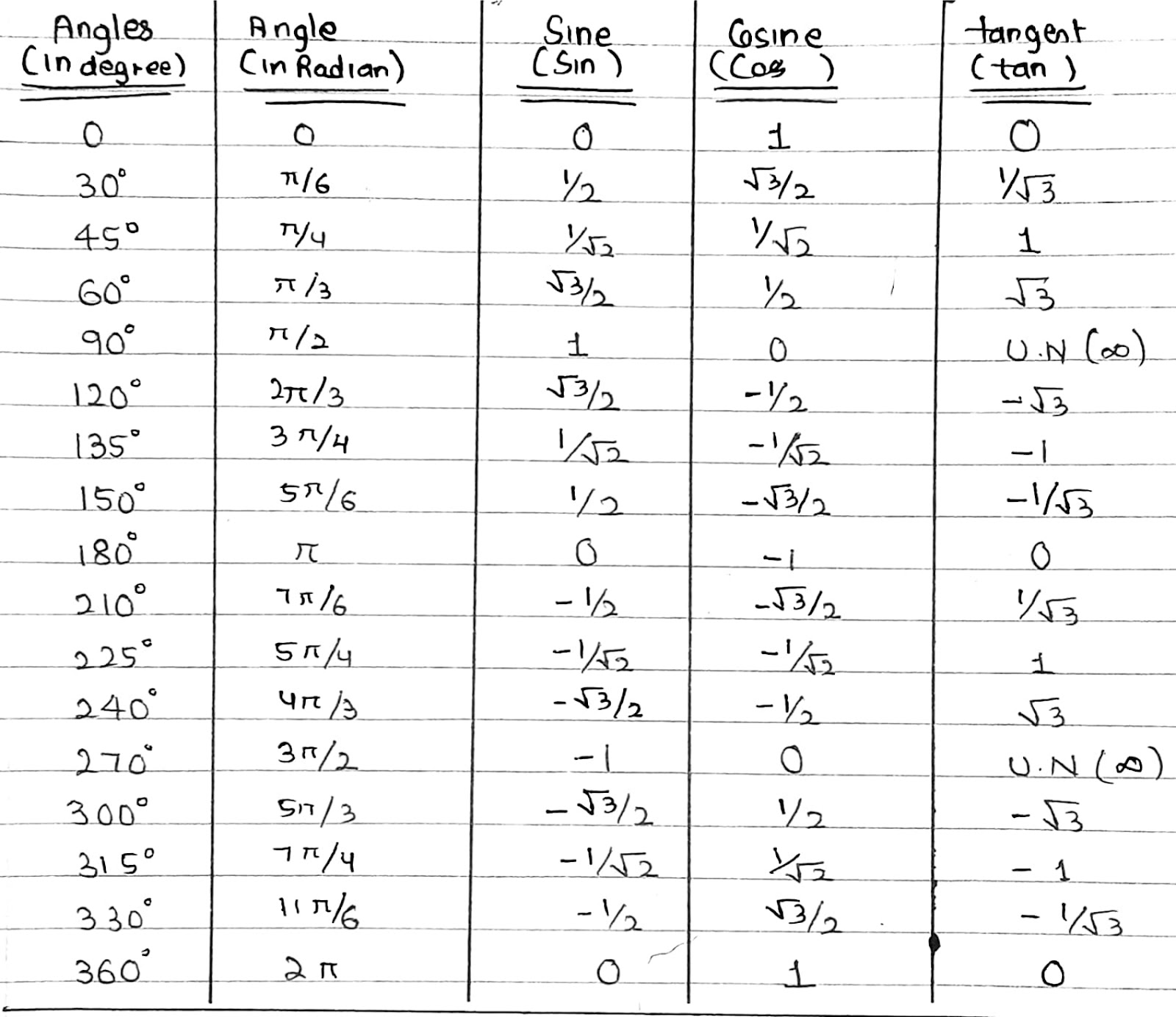
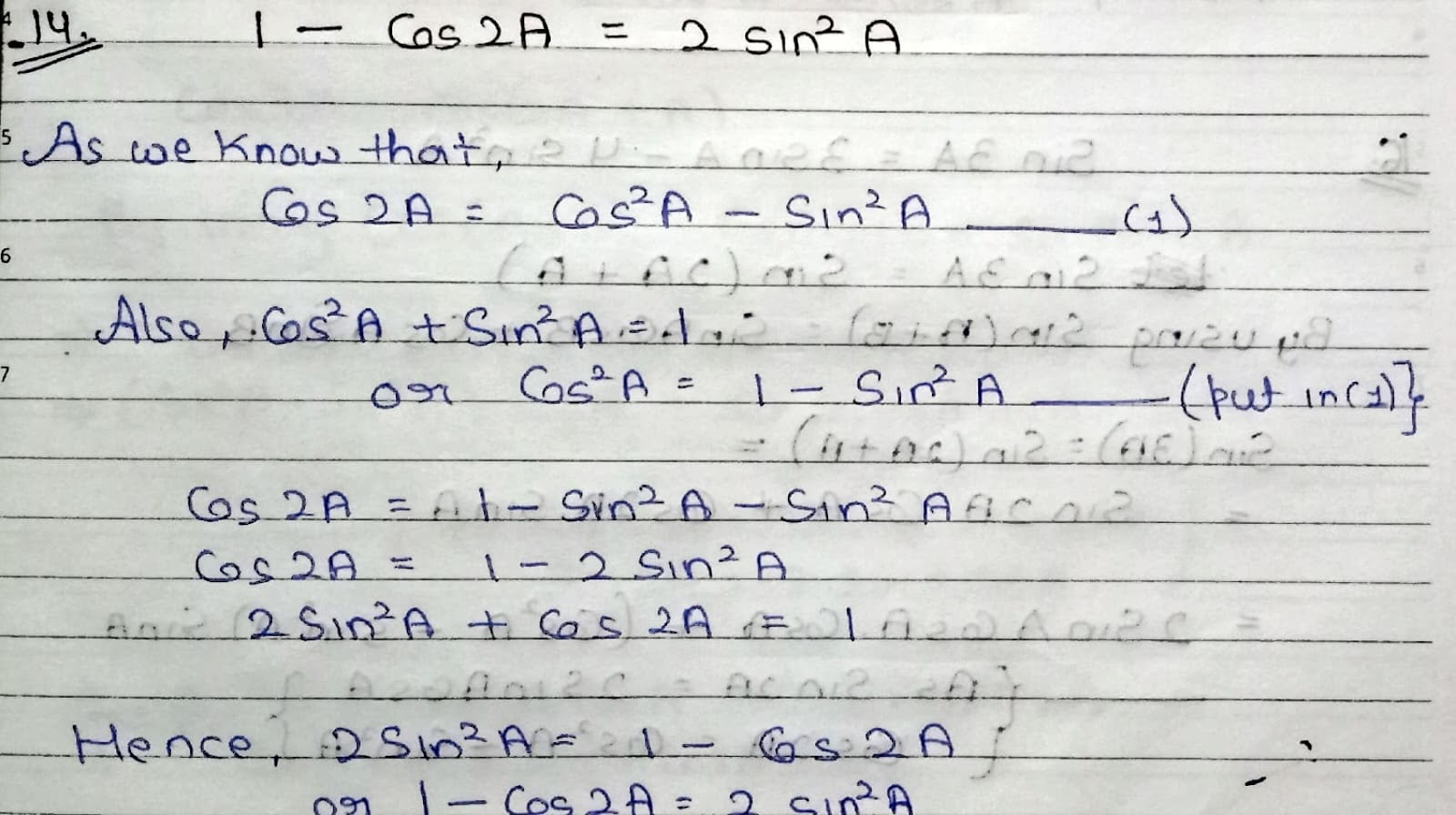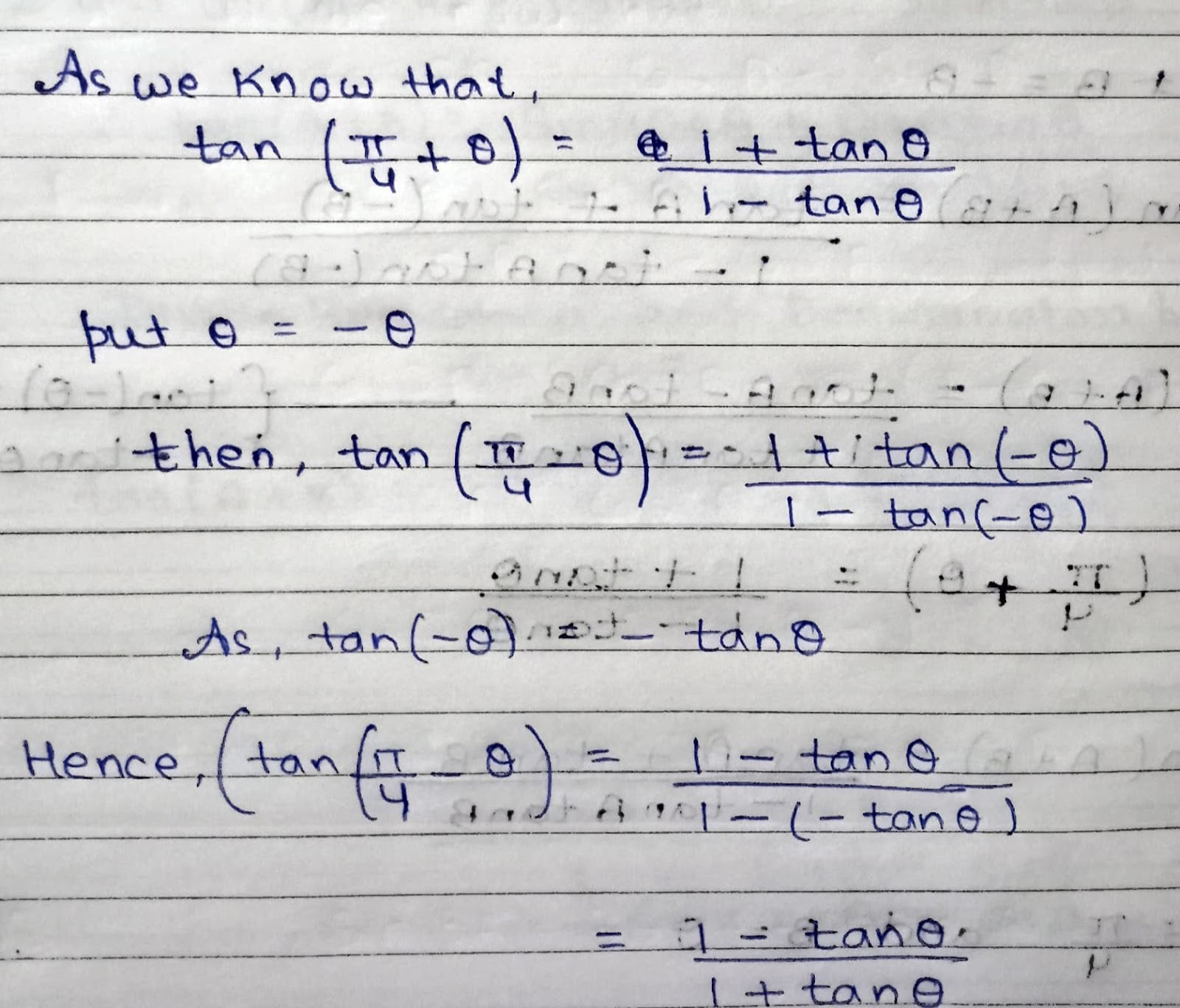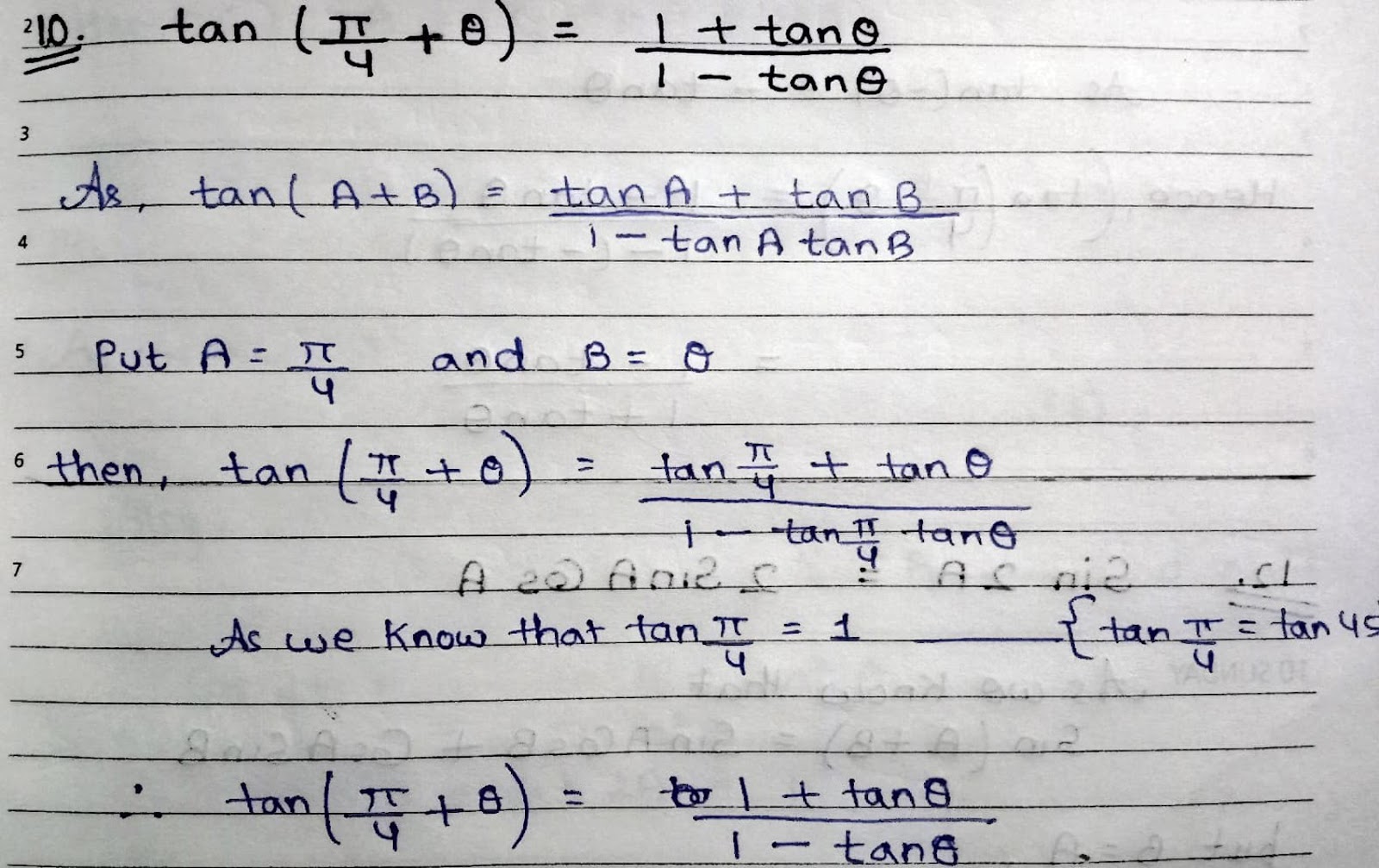How Sin (A+B) × Sin (A-B) = Sin²A - Sin²B ?

How Sin (A+B) × Sin (A-B) = Sin²A - Sin²B ? ➡ Sin(A+B) = SinA CosB + CosA SinB ➡ Sin(A-B) = SinA CosB - CosA SinB
I'm here with derivation of all the Formulae of maths. Follow me Stay tuned